When an electromagnetic wave propagates from the source, it transfers energy to objects in its path.

An electromagnetic wave stores energy in the electric and magnetic fields.
You can find the energy density of an electromagnetic wave by calculating the sum of the electric field energy density and magnetic field energy density.
An oscillating electric field generates an oscillating magnetic field, and an oscillating magnetic field generates an oscillating electric field. Electric and magnetic fields are physically inseparable and they co-exist in electromagnetic waves.
When an electromagnetic wave propagates from the source, it transfers energy to the objects in its path. An electromagnetic wave stores energy in the electric and magnetic fields. The total energy stored in an electromagnetic wave is equal to the sum of energy stored in the electric and magnetic fields. In that case, the energy stored per unit volume, or energy density of the electromagnetic wave, is the sum of the electric field energy density and magnetic field energy density.
Let’s take a closer look at electromagnetic waves and their energy density.
1、Calculating the Energy Density of Electromagnetic Waves
Consider an electromagnetic wave traveling in the free-space in the positive x-direction. The electric field associated with the wave is changing in the y-direction and the magnetic field is alternating in the z-direction. Let the electric and magnetic fields be mathematically represented as:
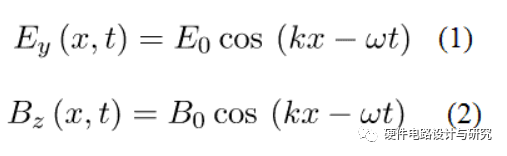
The energy stored in any part of the electromagnetic wave is the sum of electric field energy and magnetic field energy. The total energy stored per volume is the energy density of the electromagnetic wave (U), which is the sum of electric field energy density (UE) and magnetic field energy density (UB). Equation (3) gives the expression for the energy density of an electromagnetic wave, where 𝜇0 is the permeability of free space and 𝜺0 is the permittivity of free space. The unit of energy density is Joule/m3:
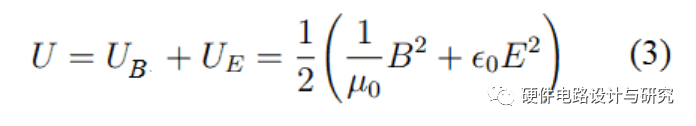
2、Expressing Energy Density in Terms of Either the Electric Field or Magnetic Field
In electromagnetic field theory, the ratio of the electric field to the magnetic field gives the speed (c) of the electromagnetic waves in free space. It can be written as follows:
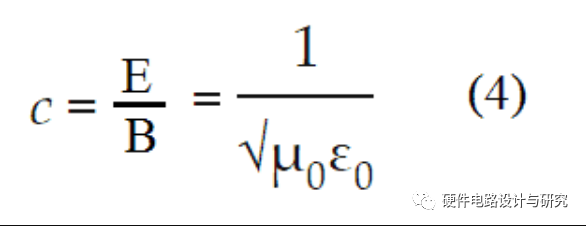
The expression in equation (4) implies that in an electromagnetic wave, the energy associated with the electric field is equal to the energy associated with the magnetic field. Hence, the energy density (U) of an electromagnetic wave can be expressed only in terms of either the electric field or magnetic field.
Equations (5) and (6) give the expression for the energy density of electromagnetic waves in terms of the electric field and magnetic field, respectively:
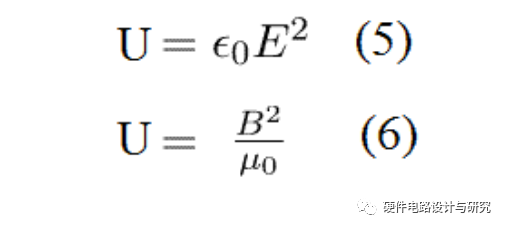
From equations (3), (5), and (6), we can summarize that in a given volume, the electromagnetic energy is shared equally between the electric and magnetic fields.
3、The Relationship Between the Poynting Vector, Wave Intensity, and Energy Density
The Poynting vector S is the rate at which electromagnetic energy flows through a unit surface area perpendicular to the direction of propagation of the wave. Basically, it is the rate of flow of energy in an electromagnetic wave, given by equation (7):
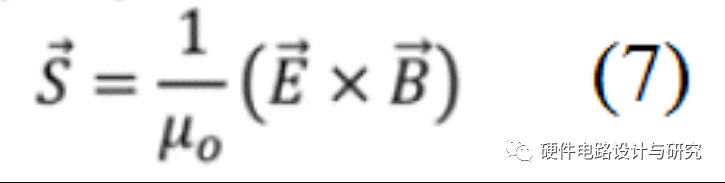
Like the electric field and magnetic field, the magnitude of the Poynting vector S also varies with time. The maximum values of E, B, and S occur at the same instant. However, the S vector is oriented towards the direction of propagation of the wave. The unit of the Poynting vector is W/m2 which indicates it is the electromagnetic power per unit area.
It is important to remember that S is time-varying. In such quantities, the average value is of great importance. The term wave intensity (I) gives the average time of the Poynting vector S and can be denoted as Savg. The relationship between the Poynting vector, wave intensity, and energy density can be given by the following equations:
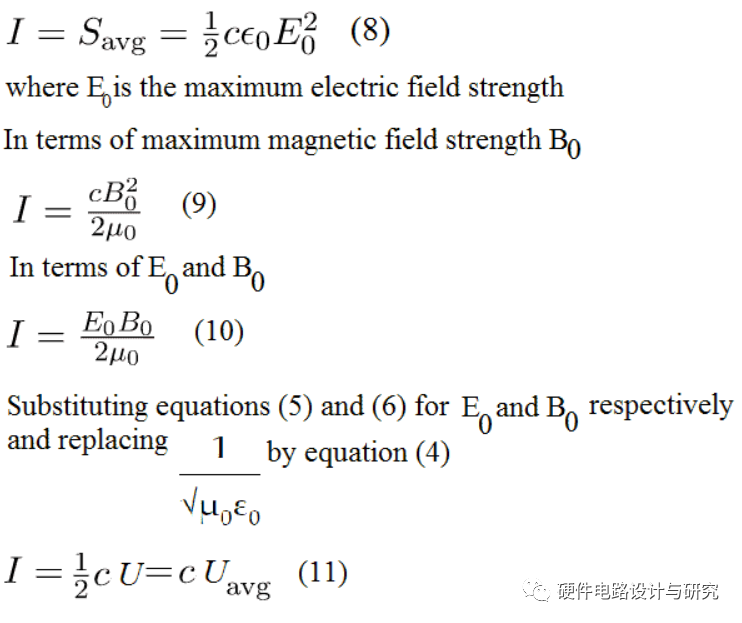
The energy density of an electromagnetic wave is completely dependent on the electric and magnetic fields of the wave. By using these calculations, you can determine the energy density of an electromagnetic wave.